How to Find Expected Value(Utimate use of Expected Value Formula)?
In this article, you will learn about expected value probability. You will also learn the expected value formula and how it is calculated.
What is Expected Value?
It is exactly what you might think about it, which is the expected result of some action or calculations. In statistics, the expected value is also known as the mean value. It is a long-run average value of random variables. It is also known as the probability-weighted average of all possible outcomes.
Expected value is a concept of statistics that are highly used in finance. It indicates the anticipated value of an investment in the future. So, we can determine the possible results of our investment with it.
Expected Value Formula
The expected value probability formula of an event is obtained by multiplying the sum of its probability by the number of times the event is happening. EV denotes it, that is:


It gives a quick insight into the behaviour of a random variable without knowing if it is discrete or continuous.
How to find Expected Value?
Suppose for Xi number of events and probability PXi, the expected value of the event X is calculated as:
EV = Σ xi P(xi)
The expected value of a random variable is calculated by multiplying the sum of its probability and the number of possible outcomes. Here we will provide you a step-wise method of calculating expected value. These steps are:
- Construct a table by using random variable X.
- Add a column of PXi in the table by finding the probability of all values of random variables.
- Add another column of P(Xi)Xi.
- Find the sum P(Xi)×Xi that is the expected value of X.
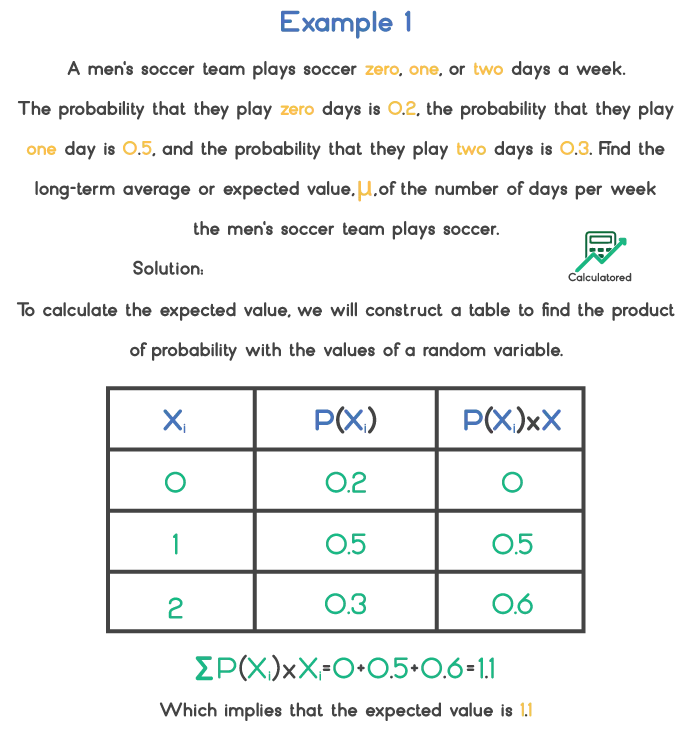
Expected Value Examples and Solutions



Related Formulas
There are three formulas that are related to expected value. These are:
- Probability Formula
- Variance Formula Expected Value
- Mean Formula
Probability Formula
It is defined as the ratio of favourable outcomes of an event and all outcomes. The probability formula of an event A is:
$$ P(A) \;=\; \frac{n(A)}{s(A)} $$Where,
nA=favourable outcomes
nS= total outcomes of A.
Variance Formula Expected Value
The variance is the expected value of the squared variation of a random variable from its mean. The variance is also calculated with the expected value of the random variable. The formula is:
$$ σ^2 \;=\; Σ(x-μ)^2 \; P(x) $$Where,
=mean
Px=probability of the event
Mean Formula
The mean of a random variable x is defined as the sum of its all values by the total number of values. The mean formula is:
$$ \bar X \;=\; \frac{ΣX}{n} $$The mean is also defined in terms of expected value that is:
$$ μ \;=\; P(X_i) \;×\; X_i $$FAQ’s on Expected Value Formula
Is the Expected Value the Same as Mean?
The mean is used when we want to calculate the average of a sample data while the expected value is used when we want to calculate the mean of a probability distribution.
What is the Expected Value in Math?
It is a long-run average value of random variables. It is also known as the probability-weighted average of all possible outcomes. It is also referred to as the mean of a probability distribution.


