What is Factorial Formula? How do you solve Factorials?
This article will learn what factorial is and how it is calculated. You will also learn how double factorial is calculated.
What is Factorial?
The term factorial of a number is defined as the product of that number with the previous numbers. The symbol represents it “!”. The factorial can be calculated for all whole numbers by multiplying all previous natural numbers. In mathematical analysis, factorial is a key to approaching other mathematics subjects like algebra, number theory, probability theory, and computer science. It is also used in exponents and power series.
Factorial Formula
The factorial of a whole number n is calculated by multiplying all previous natural numbers. The factorial formula of n can be written as:

n×(n-1)×(n-2)×...1 = the product of all previous natural numbers.
Let’s calculate the factorial of 5 using the following steps.
Step#1:We will write the factorial of 5 in its symbolic form as 5!
Step#2:Now compute the product of 5 with its previous natural numbers.
5!=5×4×3×2×1
5!=120
How do you Solve Factorial Questions?
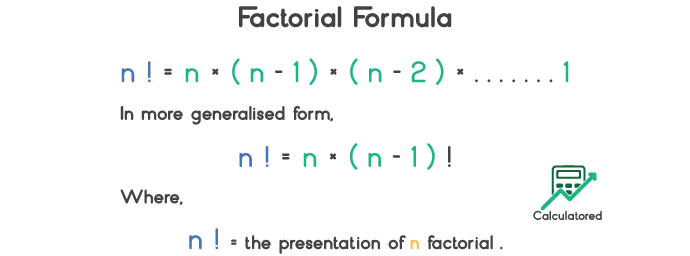
Let n be a whole number, to calculate n! We have to multiply n by its previous natural numbers such that:
n!=n×(n-1)×(n-2)×...1
Where,
n!= the presentation of n factorial.
n×(n-1)×(n-2)×...1 = the product of all previous natural numbers.
Let’s see the following examples to calculate factorial.


Double Factorial Formula
The double factorial of a number is the product of all integers up to 1. If the number is even then its factorial is the product of all even numbers less than that number. And if the number is odd then its factorial is the product of all odd numbers. The double factorial formula is given as:
n‼ = n×(n-2)×(n-4)×...×2×1
If n is even,
n‼ = n×(n-2)×(n-4)×...×2
If n is odd,
n‼ = n×(n-2)×(n-4)×...3×1
Let’s see the following example to calculate double factorial.
Example: what is the double factorial of 5?
Since 5 is an odd number using the double factorial formula for odd integers.
5‼ = 5×3×1
5‼ = 15
Related Formulas to Factorial
There are two formulas related to factorials. These are:
- Permutation Formula
- Combination Formula
Permutation Formula
The permutation of a set of numbers can be defined by representation of the set in the possible arrangements. The permutation formula is:
$$ ^nP_r \;=\; \frac{n!}{(n-r)!} $$
Where P represents permutation of n and r denotes the number of selected elements from the set.
Combination Formula
The combination of a set of numbers can be defined by the representation of the numbers in possible collection of the arrangements. The combination formula is:
$$ ^nC_r \;=\; \frac{n!}{r!(n-r)!} $$
Where C is a combination term of n and r be the selected number.
FAQ’s
What is the Formula for Factorial?
The factorial formula of a whole number n is:
n!=n×n-1!
What is the Factorial of 0?
If we use n=1 in the factorial formula as:
1!=11-1!
Since the factorial of 1 is 1.
1=10!
1=0!


