Understand the Concept of Integration Formulas & How to Evaluate Integrals?
In this article, you will learn what integration is and how to calculate integrals. You will also learn what are the basic rules/formula of integration.
What is Integration?
In mathematics, integration refers to the opposite of derivative. For example if the derivative of function fx is gx, then the integration of gx will be the original function fx. The integration is denoted by ∫. Since the derivative is the rate of change of a function and the integration is the inverse of differentiation.
In mathematics, derivative and integration are two major factors that help to find the rate of change of a function (derivative) and region bounded by a curve. It is done by splitting the curve into infinitesimal small areas and adding them all together. These small areas can be referred to integrals and the process of finding integrals is called integration.
Integration Formula
The formula of integration for a function f(x) can be written as:


Since we already know the formulas of derivatives, we can use them to derive basic integration formulas. For example, the derivative of f(x)=x is f’(x)=1 so to derive formula for integration of f(x),
$$ f'(x) \;=\; \frac{dy}{dx} \;=\; 1 $$ $$ \int f'(x)dx \;=\; \int \; 1dx \;=\; x+c $$Similarly if we have,
$$ f'(x) \;=\; cos(x) $$The integration is:
$$ \int f'(x) dx \;=\; \int \; cos(x) dx = sinx\;+\;c $$Here sinx is the original function and cosx is its derivative. If we reverse the derivative into the original function, the resulting function is called integration.
Similarly, there are also some special integrals formulas that are commonly useable while evaluating integrals. These other formulas for integration of different functions are divided into following categories:
- Polynomial formulas
- Integration Trigonometry Formulas
- Inverse trigonometry formulas
- Exponential formulas
- Hyperbolic formulas
Polynomial Formulas
$$ \int x^n dx \;=\; \frac{x^{n+1}}{n+1} \;+\; C $$ $$ \int dx \;=\; x \;+\; C $$Integration Trigonometry Formulas
$$ \int cos(x)dx \;=\; sin(x)+c $$ $$ \int sin(x)dx \;=\; -cos(x)+c $$ $$ \int sec^2(x)dx \;=\; tan(x)+c $$ $$ \int cosec^2(x)dx \;=\; -cot(x)+c $$ $$ \int sec(x) \; tan(x)dx \;=\; sec(x)+c $$ $$ \int cosec(x) \; cot(x)dx \;=\; -cosec(x)+c $$Inverse Trigonometry Formulas
$$ \int \frac{dx}{\sqrt{1-x^2}} \;=\; sin^{-1}(x) + c $$ $$ \int -\frac{dx}{\sqrt{1-x^2}} \;=\; -cos^{-1}(x) + c $$ $$ \int \frac{dx}{1+x^2} \;=\; tan^{-1}(x) + c $$ $$ \int -\frac{dx}{1+x^2} \;=\; cot^{-1}(x) + c $$Exponential Formulas
$$ \int e^x dx \;=\; e^x $$ $$ \int e^{-x} dx \;=\; -e^{-x} $$ $$ \int e^{ax} dx \;=\; \frac{1}{a} e^{ax} $$ $$ \int x \; e^{ax} dx \;=\; \frac{e^{ax}}{a^2} (ax-1) $$ $$ \int x^m \; e^{ax} dx \;=\; \frac{x^m \; e^{ax}}{a} \;-\; \frac{m}{a} \int x^{m-1} \; e^{ax} dx $$ $$ \frac{1}{x} \; e^{ax} \;=\; ln(x) \;+\; \frac{ax}{1!} \;+\; \frac{a^2 \; x^2}{2 \cdot 2!} \;+\; \frac{a^3 \; x^3}{3 \cdot 3!} \;+\; \frac{a^4 \; x^4}{4 \cdot 4!} \;+\; ... $$ $$ \int xe{-x^2} dx \;=\; -\frac{1}{2} e{-x^2} $$Hyperbolic Functions
$$ \int cosh(x) dx \;=\; sinh(x)+c $$ $$ \int sinh(x) dx \;=\; cosh(x)+c $$ $$ \int sech^2(x) dx \;=\; tanh(x)+c $$ $$ \int cosech^2(x) dx \;=\; -coth(x)+c $$ $$ \int sech(x) \; tanh(x) dx \;=\; -sechh(x)+c $$ $$ \int cosech(x) \; coth(x) dx \;=\; -cosechh(x)+c $$Properties of Integration
The basic properties of integration are:
$$ \int (af(x))dx \;=\; a \int f(x)dx $$ $$\int (f(x)+g(x))dx \;=\; \int f(x)dx + \int g(x)dx $$Interval < a < b < c
$$ \int_a^c f(x)dx \;=\; \int_a^b f(x)dx + \int_b^c f(x)dx $$ $$ \int_a^b\;f(x)dx \; = \; - \int_b^a f(x) dx $$ $$ \int_a^b \;f(x)dx \; = \; f(b) \;-\; f(a)$$which is the fundamental theorem of calculus.
How to Evaluate Integrals?
We can calculate the integration of a function by using its derivative. The fundamental theorem of calculus (special integrals formula) helps us to find the integral of a function that is:
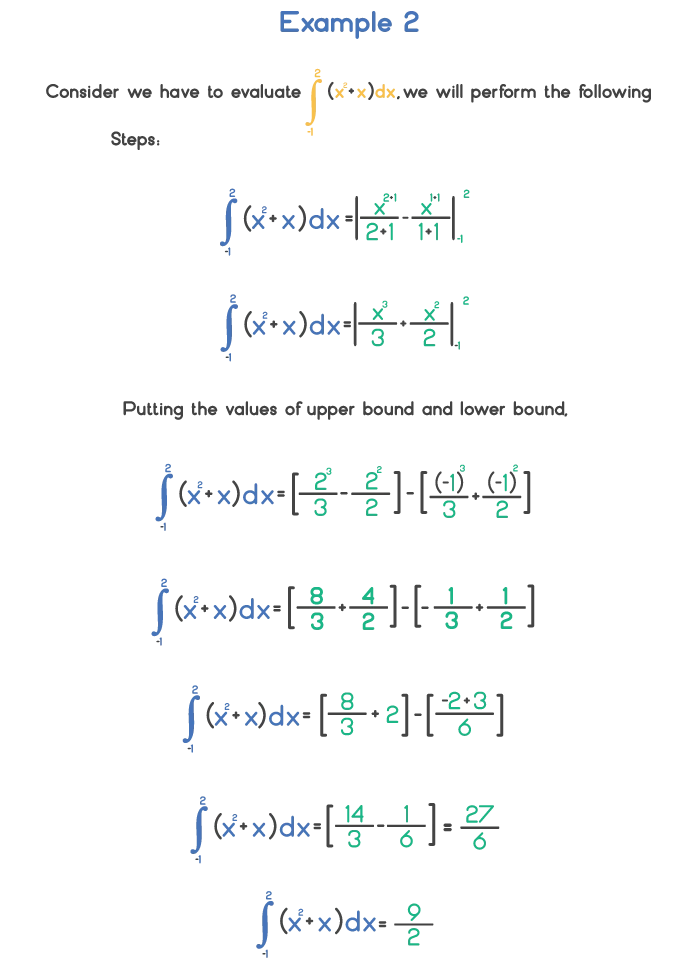
$$ \int_(a)^(b) \; f(x)dx \;=\; f(b) - f(a) $$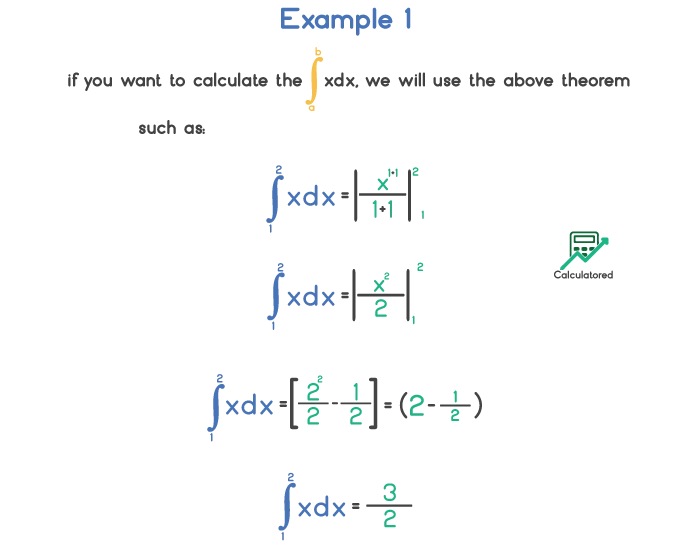


FAQ’s
What is Integration?
Integration is a technique of finding area under the curve by finding the inverse of differentiation.
How do you Integrate the Area of a Sector?
The area of a sector of a circle depends upon the area of the triangle with some additiional curved section. The formula to calculate the area of a sector with integral calculus is:
$$ \int_{r cos\theta}^r \sqrt{r^2 - x^2} dx $$Why do we Study Integration?
To understand the direct relation between two variables, we need to study integration.
How are limits and integrals related?
In the integral calculus, definite integrals to find the exact area under the curve and the Rieman sum helps to estimate the area under the curve. To take the precise area under the curve, we have to take the Riemann sum for infinite rectangles of infinitely small width. This is only possible with the help of the concept of limits.


