Understanding the Z Score Formula & It's Calculation with Examples
In this article, you will learn what a z-score is and how it is calculated. You will also learn how it is related to mean.
What is Z-Score?
In statistics, the z-score is the measure of distance between an observation point and the mean value using standard deviation. Z-scores can have positive and negative signs. The positive sign indicates that the data point falls standard deviation above the mean and the negative sign indicates that the data point falls standard deviation below the mean. The z-score of any observation tells whether it falls above or below the mean.
The z-score is also known as the standard score. It allows finding the probability of a data point in a normal distribution. It helps to choose a sample value that is near to the mean of the sample distribution.
Z Score Formula (Standard Score Formula)
The z-score tells the variation of a date point whether it lies above or below the sample's mean. The distance between the mean and a sample data point is calculated by using standard deviation. The following formula is used to calculate the z-score or standard score of a data point:
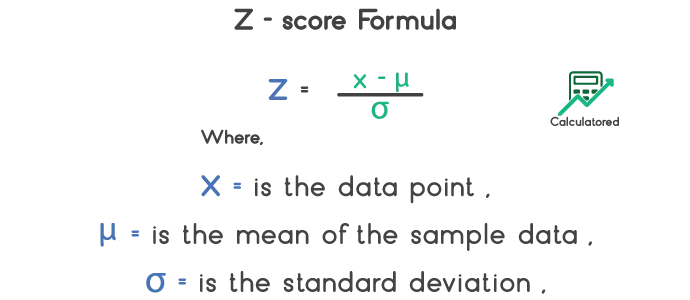

For example, the z-score of a data point 2 with the sample mean 10 and standard deviation 2 can be calculated as:
$$ z \;=\; \frac{2-10}{2} $$ $$ z \;=\; \frac{-8}{4} \;=\; -4 $$
Here, Z=-4 indicates that the sample data point 2 falls 4 steps below the mean.
How do you calculate the z-score?
If x is a data point, μ is mean (obtained by using mean math formula) and σ is the standard deviation of a population then the standard score can be calculated by the following formula:
$$ Z \;=\; \frac{x-μ}{σ} $$


It means that the sample point 10 lies zero standard deviations away from the mean.
Advantages of Z-score
Z-score is an essential term in statistics that has the following advantages:
- The z value formula helps find the probability of a data point so that we can select that sample point near the mean.
- It helps to compare two data points of a normal distribution.
- It is a financial tool that helps to optimise profit and loss.
Related to Z Formula
The Z score formula is the main formula in statitics, the other relevant formulas to z score are:
- Probability in Statitics $$ P(A) \;=\; \frac{n(A)}{n(S)} $$
- Artirhmetic Sequence Formula $$ a_n \;=\; a_1 + (n-1)d $$
- Geometric Sequence Formula $$ S_n \;=\; a \frac{(1-r^n)}{1-r} $$
FAQ’s
What does z-score mean?
The z-score indicates how much far or near is the given data point from the mean. It is the distance between population mean and the data point.
What is the Z Formula?
The z formula is used to calculate the z-score of a data point by using the population mean and standard deviation. The formula is:
$$ Z \;=\; \frac{x-μ}{σ} $$
How do you find the z-value in a normal distribution?
The knowledge of the population mean and the standard deviation is required to find the z-value in a normal distribution. The z-score value can be calculated using both mean and standard deviation in the z-score formula.


