What is A Circumference of Circle Formula ? How to calculate the circumference of a circle?
In this article, you will learn what the circumference of a circle is. You will also understand the circumference formula.
What is A Circumference of a Circle?
The circumference of a circle measures the total length of the boundary. In other words, the length of the circular path of a circle is its circumference. A circle is a two-dimensional shape that has area and perimeter. The circumference, also known as the circle's perimeter, is measured in units such as cm or m.
In mathematics, the perimeter and area of a circle are important factors in describing the properties of the circle. Here you will understand the formula of circumference and its calculations.
Circle Circumference Formula
The circumference of a circle is the length of its circular path or boundary. It depends on the radius of the circle. The formula for circumference is:
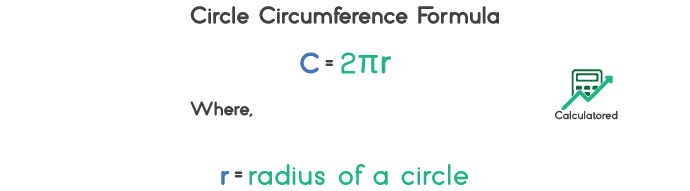

Let’s understand the concept of circumference with an example.
If a boy starts running in a park from the starting point A and completes a round by running along the circular path of the park. Then the total distance covered by the boy is the circumference of the park.
How is the Formula for Circumference Derived?
By the definition of (pi) π, It is the ratio of circumference and diameter of a circle. So,
$$ \pi \;=\; \frac{c}{d} $$By arranging above equation,
$$ C \;=\; \pi d $$As we know, the diameter of a circle is twice of its radius so we have,
$$ C \;=\; 2 \pi r $$Which is the formula to find the circumference of a circle.
How to Find the Circumference of a Circle?
To find the circumference of a circle, the radius of the circle should be known. Consider the radius of a circle is r then the circumference formula is:
C = 2πr
See the following examples to understand how the circumference is calculated by using the circumference of circle formula.


Area of a circle with Circumference
The area of a circle is referred to the total space occupied by the circle. The area of a circle can be calculated by using area formula and circumference also. The formula for calculating the area of a circle with circumference is:
$$ A \;=\; \frac{C^2}{4 \pi} $$Where,
C = circumference of the circle
So if the circumference of a circle is known, we can calculate the area by using the above formula.
Let’s see the following example to understand how we can calculate the area of a circle with circumference.


Related Formulas
- Area of a Sector Formula
- Arc Length Formula
- Volume of Sphere Formula
Area of a Sector Formula
The sector is is the part of a circle enclosed with boundary lines (the radii of circle). The formula of sector area is:
$$ A \;=\; \frac{θ}{360} \pi r^2 $$Arc Length Formula
The arc length is the distance of curved line between two points. To find the curved line length of a circle, we may use length of an arc formula. This formula is as follow:
$$ L\;=\; \frac{θ}{180^\circ}×\pi r $$Volume of Sphere Formula
Volume of a sphere refers to the total occupied surface of sphere. The formula for sphere volume is:
$$ V \;=\; \frac{4}{3} πR^3 $$FAQ’s
How to calculate diameter from circumference?
We can calculate diameter of a circle using the following relation:
$$ C \;=\; \pi d $$By using the above circle circumference formula, we can calculate the circumference if the diameter is known and vice versa.
What is the circumference of a 20inch circle?
Given that, the diameter of the circle is 20inch, the circumference will be:
$$ C \;=\; \pi d $$ $$ C \;=\; \pi × 20 \;=\; 62.83inches $$

